Representation Practice
| Representation Practice | |
| Contributors | Paul Salvador Inventado, Peter Scupelli |
|---|---|
| Last modification | June 6, 2017 |
| Source | Inventado and Scupelli (2016).[1] |
| Pattern formats | OPR Alexandrian |
| Usability | |
| Learning domain | |
| Stakeholders | |
Ask students to answer practice problems that require them to use different representations so they gain experience and hone their skills in selecting, applying, and utilizing appropriate representations.
Context
Students recently started to learn about a new topic discussed in class.
Problem
Students get stuck when they don't know how to represent a problem or solution.
Forces
- Lack of prior knowledge. Students cannot apply a representation that they have not seen or used.
- Availability bias. Students are likely to use representations that they can easily recall, but these may not be appropriate to the problem they are currently solving.
- Appropriate representation. Students may know about different types of representations, but they may not know how to select what is most appropriate to a given problem.
Solution
Therefore, use an online learning system to provide students with practice problems that require them to create and use different representations. For example, one practice problem’s text may describe geometric shapes that would require students to draw the figure. Another problem may require students to recall and use an algebraic equation. Yet another problem may require students to do both.
Research shows that students learn better with frequent and spaced practice compared to massed practice. For example, give students practice problems that involve drawing isosceles triangles twice a week and mix it with problems that involve other representation types.
Students who have just started to learn using a particular representation might have a difficult time to answer practice problems. Providing students with worked examples and interleaving them with practice problems have been found to be effective. As students gain expertise worked examples can be faded so that they answer practice problems themselves.
The Represent It design pattern can help to encourage students use representations.
Consequences
Benefits
- Practice problems give students opportunities to learn about many different representations.
- Practice helps to build memory and fluency, which facilitates the recall of different representations.
- As students practice on different representations, they begin to learn when, why, and how to apply them.
Liabilities
- Teachers need to design and implement various practice problems on the online learning system for students to learn from.
- Students who continuously fail to answer problems may lose motivation. They may be more likely to give up when asked to solve similar problems. Worked examples or feedback may be needed to support learning.
- It takes time for students to learn and master skills for representing problems and solutions.
Evidence
Literature
- Practice promotes deeper understanding of skills that integrate with prior knowledge to develop expertise[2]. Experts from different domains devote significantly more time to practice compared to average and novice performers.
- Practice requires students to recall and apply information, which leads to better learning compared to instruction alone[2]. Frequent, but spaced practice leads to better learning because it allows students to forget information, but requires them to recall information in the next practice session[2][3].
- Students who are just starting to learn a particular topic may lack prior knowledge to answer practice problems. An effective strategy is to interleave worked examples and practice problems so that students learn how to perform a skill and get practice applying it themselves then gradually decrease examples and increase practice to develop students’ skills[4].
Related patterns
The Representation Practice design pattern implements the Try It Yourself design pattern[5], whose goal is to promote better understanding of a topic through exercises, but in the context of representing math-problems. Consider using the Image-Enhanced Problem design pattern to clearly express problems with confusing or ambiguous elements.
Students may still get stuck answering a problem if they make incorrect representations or if they are unable to make the correct inferences. The Represent It design pattern may help guide the student to make and interpret representations. Hints may help keep students motivated to answer the problem as described in the Build and Maintain Confidence design pattern[5]. Create a diverse set of problems as described in the One Concept Several Implementations design pattern[5] so that students are exposed to various problems that require different representations to solve.
Example
Math teachers use notations, equations, and figures to clarify concepts they teach and encourage students to apply the same strategy when they solve practice exercises (e.g., McIntosh 2015[6], Noh et al. 2013[7], Polya 2014[8]). Math online learning systems such as ASSISTments[9], Cognitive Tutor Geometry[10], and Picture Algebra[11] also provide students with practice problems that contain many problem details, which students need to represent correctly to solve.
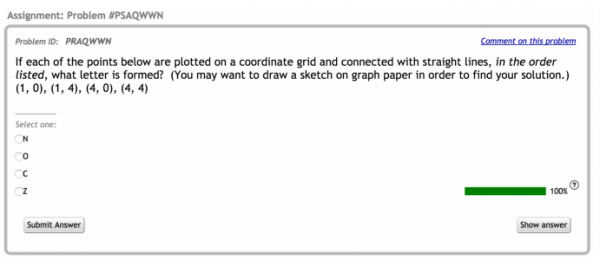
The following figure shows an example of a practice problem for “Understanding the Coordinate System” in the ASSISTments online learning system. It may be difficult to solve the problem mentally, so it encourages the student to draw a figure.
References
- ↑ Inventado, P.S. and Scupelli, P. (2016). Design Patterns for Helping Students to Learn to Visualize Math Problems in Online Learning Systems. In Proceedings of the 21st European Conference on Pattern Languages of Programs (EuroPLoP 2016). New York:ACM.
- ↑ 2.0 2.1 2.2 Clark, R. C., & Mayer, R. E. (2016). E-learning and the science of instruction: Proven guidelines for consumers and designers of multimedia learning. John Wiley & Sons.
- ↑ Rohrer, D., & Taylor, K. (2006). The effects of overlearning and distributed practise on the retention of mathematics knowledge. Applied Cognitive Psychology, 20(9), 1209-1224.
- ↑ Renkl, A. (2014). The worked examples principle in multimedia learning. In R.E. Mayer (Ed.), The Cambridge handbook of multimedia learning (pp. 391–412). New York: Cambridge University Press.
- ↑ 5.0 5.1 5.2 Bergin, J., Eckstein, J., Völter, M., Sipos, M., Wallingford, E., Marquardt, K., Chandler, J., Sharp, H., and Manns, M.L. (2012). Pedagogical patterns: advice for educators. Joseph Bergin Software Tools.
- ↑ McIntosh, K.L. (2015). Montessori Mathematics Curriculum and Lower Elementary Students Understanding of Length Measurement. Masters of Arts in Education Action Research Papers. Paper 148.
- ↑ Noh, J., Warren, J., Huh, N., & Ko, H. K. (2013). Instruction Using Scaffolding for Language Learner Students in Solving Mathematical Word Problems. Research in Mathematical Education, 17(3), 169-180.
- ↑ Polya, G. (2014). How to solve it: A new aspect of mathematical method. Princeton university press.
- ↑ Heffernan, N.T. and Heffernan, C.L. (2014). The ASSISTments Ecosystem: Building a Platform that Brings Scientists and Teachers Together for Minimally Invasive Research on Human Learning and Teaching. International Journal of Artificial Intelligence in Education 24(4), 470-497.
- ↑ Aleven, V., Mclaren, B., Roll, I., & Koedinger, K. (2006). Toward meta-cognitive tutoring: A model of help seeking with a Cognitive Tutor. International Journal of Artificial Intelligence in Education, 16(2), 101-128.
- ↑ Koedinger, K. R. (2002). Toward Evidence for Instructional Design Principles: Examples from Cognitive Tutor Math 6. In Proceedings of the Annual Meeting [of the] North American Chapter of the International Group for the Psychology of Mathematics Education, ERIC/CSMEE Publications, Columbus, OH, USA, pp. 21-49.